SOLUTION Here only tan x occurs, so we use tan 2x sec 2x 1 to rewrite a tan 2x factor in terms of sec 2x y tan x dx y tan x tan x dx 3 2 y tan x sec 2x 1 dx y tan x sec 2x dx y tan x dx tan 2x 2 ln sec x C In the first integral we mentally substituted u tan x so that du sec 2x dx2 The answer is this but the coefficient is 1 4 Why? · If \(k\) is even and \(j\) is odd, then use \(\tan^2x=\sec^2x−1\) to express \(\tan^kx\) in terms of \(\sec x\) Use integration by parts to integrate odd powers of \(\sec x\) Example \(\PageIndex{8}\) Integrating \(∫\tan^kx\sec^jx\,dx\) when \(j\) is Even
Integral Of Sec 6 X Tan 2 X Dx
Tan^2x secx integral
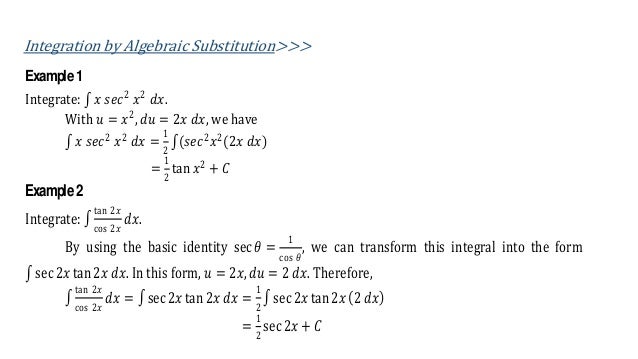
Tan^2x secx integral-Evaluate integral of 1/(sec(x)^2) with respect to x Simplify Tap for more steps Rewrite as Rewrite as Rewrite in terms of sines and cosines Multiply by the reciprocal of the fraction to divide by Multiply by Use the halfangle formula to rewrite asSubsection 1 Integrating \(\int \tan^m x\sec^n x\dee{x}\) The strategy for dealing with these integrals is similar to the strategy that we used to evaluate integrals of the form \(\int \sin^m x\cos^n x\dee{x}\) and again depends on the parity of the exponents \(n\) and \(m\text{}\)
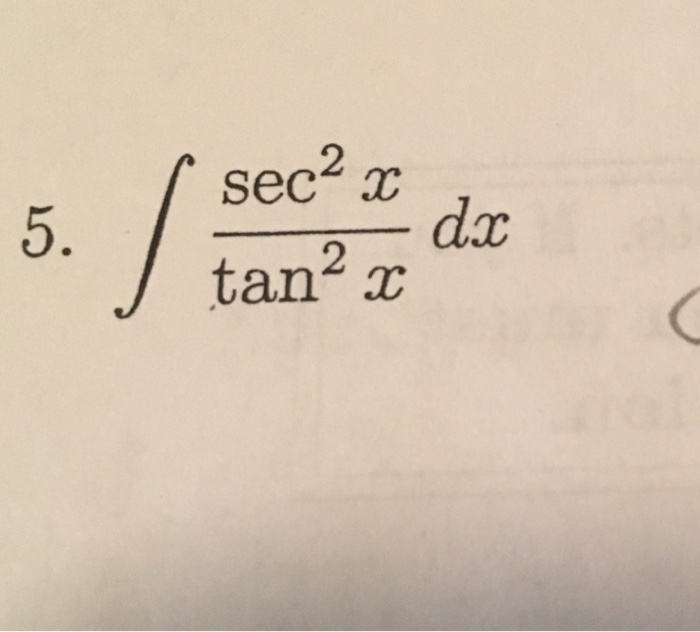


Solved Integral Sec 2 X Tan 2 X Dx Chegg Com
The Integral Calculator supports definite and indefinite integrals (antiderivatives) as well as integrating functions with many variables You can also check your answers! · Using properties of definite integrals, evaluate the following∫(0>π) (x tanx)/(secxtanx) dx · Integral of u^2 is NOT (u^3)/3 c Rather, integral of (u^2)du = (u^3)/3 c In (tan^2)x your 1st mistake is not writing dx Note that dx is NOT always du!!!!!
Question Find the indefinite integral (Use C for the constant of integration) I see sec 2x tan 2x dx This problem has been solved!Integrate sec^22x To integrate sec^22x, also written as ∫sec 2 2x dx, sec squared 2x, (sec2x)^2, and sec^2 (2x), we start with a u substitution Let u = 2x This is a simple u substitution Therefore du/dx = 2 This is a simple differentiation step We rearrange the previous expression for dxWe can simplify the implementation of the integral with the help of substitution, wherein we allow u= sec2x u = sec 2 x This substitution entails the change in the differential variable and the
The definite integral of from to , denoted , is defined to be the signed area between and the axis, from to Both types of integrals are tied together by the fundamental theorem of calculus This states that if is continuous on and is its continuous indefinite integral, then This means Sometimes an approximation to a definite integral is(Use C for the constant of integration) I see sec 2x tan 2x dx ;\\int \tan^{2}x\sec{x} \, dx\ > <



Integral 1 Tan 2 X Sec 2 X Youtube


Integral Of Tan 6x Sec 2x Dx
Derivatives Derivative Applications Limits Integrals Integral Applications Integral Approximation Series ODE Multivariable Calculus Laplace Transform Taylor/Maclaurin Series Fourier Series Functions Line Equations Functions Arithmetic & Comp Conic Sections TransformationSolution for Evaluate the integral S tan (2x) sec°(2x) dx Social Science AnthropologySolution for 11 Evaluate the integral tan 2x dx A In sec 21 C sec² 2x C С In cos 24 C D 2 sec 2x C В



Find The Derivative Of The Given Function Y Tan 2x 1 Cot 2x I Tried Converting The Original Function In Terms Of Sin And Cos But It Was Still Too Complicated To Be Called Simplified


Ex 7 4 9 Integrate Sec 2 X Root Tan 2 X 4 Ncert Class 12
· Write tan 3 (2x) as tan 2 (2x)tan(2x) = sec 2 (2x)1tan(2x) Now the function is Integral sec 2 (2x)1 tan(2x) sec(2x)dx Put sec(2x) = u sec(2x)tan(2x)dxUse \(\tan^2x=\sec^2x1~(=u^21)\) to replace the leftover tangents \(m\) is even or \(n\) is odd Use either \(1\) or \(2\) (both will work) The power of secant is odd and the power of tangent is even No guideline The integrals \(\ds\int\sec x\,dx\) and \(\ds\int\sec^3 x\,dx\) can usually be looked up, or recalled from memory Example 223Expert Answer Previous question Next question



Integral Sec 2 X Tan 2 X Youtube Cute766


Solved Integral Tan 2x Sec 3 X Dx Chegg Com
See the answer How do you solve this integral of 3 sec(2x1)tan(2x1)?Question How Do You Solve This Integral Of 3 Sec(2x1)tan(2x1)?I = sec 2xdx Multiplying in Nr and Dr by (sec 2xtan 2x ) I = {sec 2x(sec 2x tan 2x)/(sec 2x tan 2x)}dx Let (sec 2x tan 2x) = p then2(sec 2xtan 2x sec^2 2x)dx = dp or, sec 2x(tan 2x sec 2x)dx = 1/2dp I = (1/2)(1/p)dp I = 1/2 log p C I = (1/2) log sec 2x tan 2



What Is Integral Of Sin2x Tan2x Quora
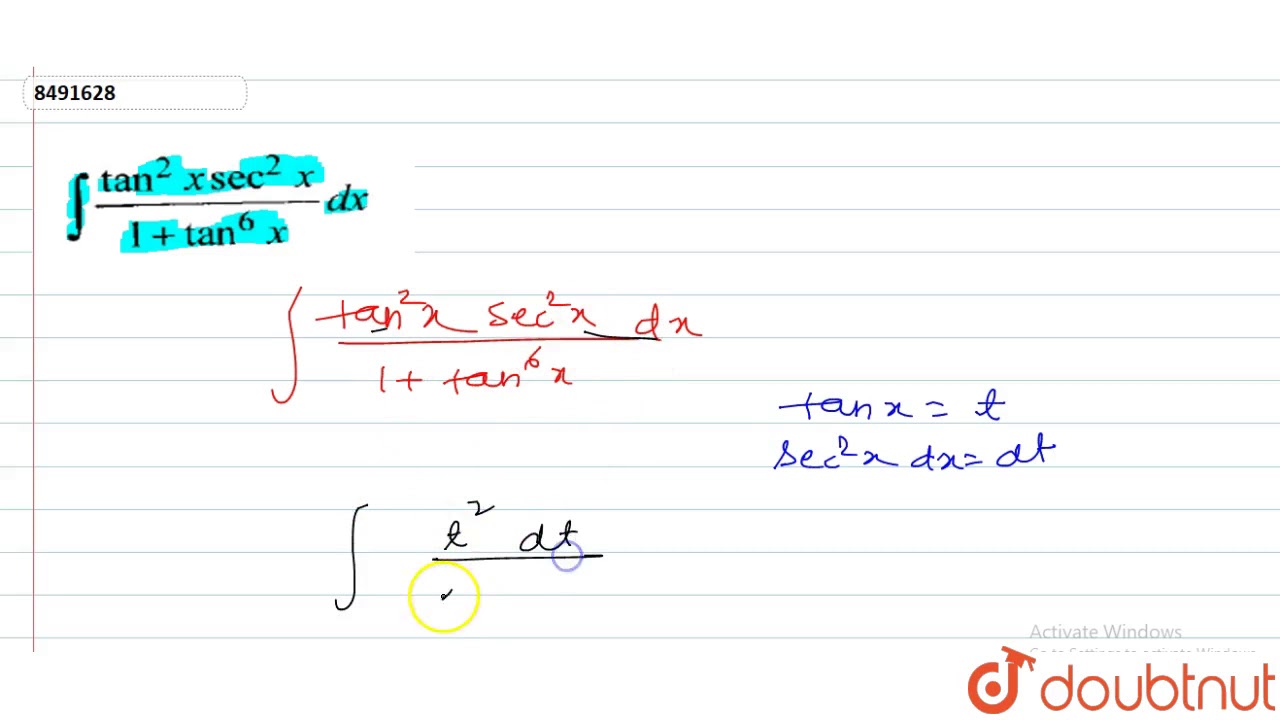


Int Tan 2xsec 2x 1 Tan 6x Dx Youtube
Integrate 2sec^2x tanx To integrate 2sec^2x tanx, also written as ∫2sec 2 x tanx dx, 2 sec squared x tan x, and 2 (sec x)^2 tanx, we start by recognising that the differential of one half is within the other half of the same expressionDifferentiate c and d, use the product rule to find v Then just use the product rule on u and v 0 · Here, notice that sec^2x is already in the integral, and all that remains is tan^2x That is, we have tanx in squared form accompanied by its derivative, sec^2x This integral is ripe for substitution!


Integrate Tan 3 2x Sec 2x Dx Sarthaks Econnect Largest Online Education Community


What Is Math Int Tan 2 2x Dx Math Quora
· In general if you vave a power of sec or tan youre gonna have to use tan^2x 1 = sec^2x and d/dx(tanx) = sec^2x and d/dx(secx) = secxtanx Yeah, that's good advice Sec/tan and cosec/cot are almost always related in the questions they give youThey use the key relations sin 2 x cos 2 x = 1 \sin^2x \cos^2x = 1 sin 2 x cos 2 x = 1, tan 2 x 1 = sec 2 x \tan^2x 1 = \sec^2x tan 2 x 1 = sec 2 x, and cot 2 x 1 = csc 2 x \cot^2x 1 = \csc^2x cot 2 x 1 = csc 2 x to manipulate an integral into a simpler formIntegration of tan^2x sec^2x/ 1tan^6x dx Ask questions, doubts, problems and we will help you Integration of tan^2x sec^2x/ 1tan^6x dx Homework Help myCBSEguide



Integral Of Sec 2x Tan 2x Integral Example Youtube
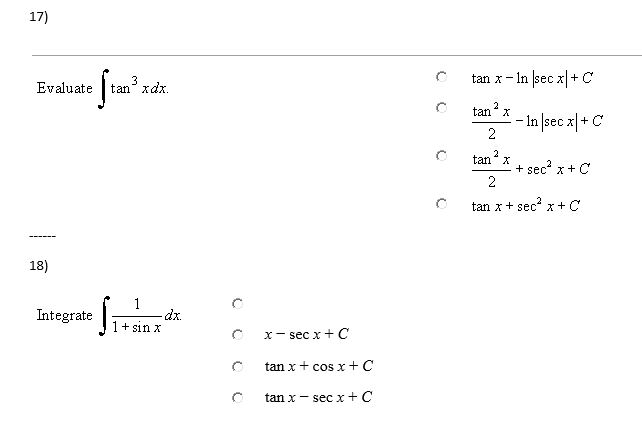


Solved Evaluate Integral Tan 3 Xdx Tan X Ln Sec X C Tan Chegg Com
In the integral inttan^2xsec^2xdx, let u=tanx and du=sec^2xdx This gives us inttan^2xsec^2xdx=intu^2du · Ex 71, 19 sec 2 2 dx sec 2 2 = 1 cos 2 1 sin 2 = 1 cos 2 sin 2 1 = sin 2 cos 2 = tan 2 = sec 2 1 = sec 2Get an answer for '`int (sec^2x)/(tan^2x5tanx6) dx` Use substitution and partial fractions to find the indefinite integral' and find homework help for other Math questions at eNotes


Integral Of Tan 4x Sec 2x



Integration Trig Identities Ppt Download
Solve the integral = ln u C substitute back u=cos x = ln cos x C QED 2 Alternate Form of Result tan x dx = ln cos x C = ln (cos x)1 C = ln sec x CIf you let u=tanx in integral (tan^2)x you get integral u^2 dx which is not (u^3)/3 c since du= sec^2x dxInteractive graphs/plots help visualize and better understand the functions For more about how to use the Integral Calculator, go to "Help" or take a look at the examples


Evaluate Sec 2 X 16 Tan 2 X Dx Sarthaks Econnect Largest Online Education Community



Evaluate Inte X Tanx Sec 2x Dx
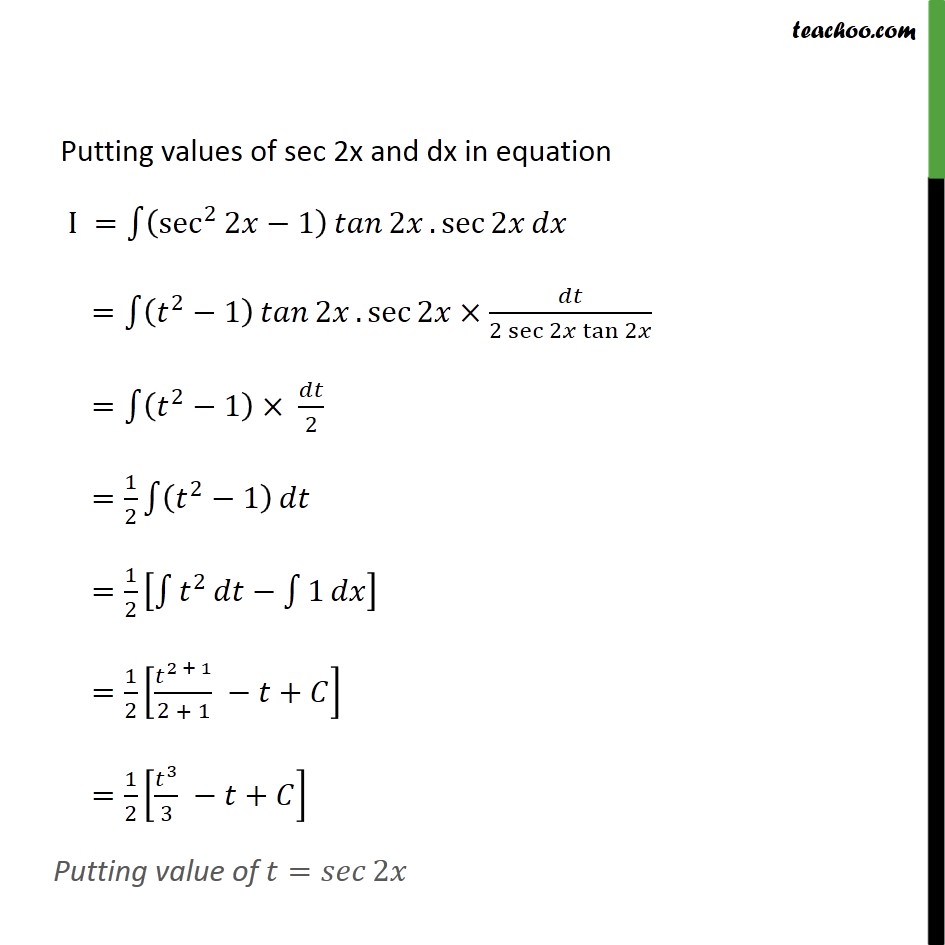
· Transcript Ex 73, 15 tan3 2 sec 2 Let I= 3 2 sec 2 = tan 2 2 tan 2 sec 2 = sec 2 2 1 2 sec 2 Putting sec 2 = Differentiating wrtx sec 2 = 2 sec 2 tan 2 = = 2 sec 2 tan 2 Putting values of sec 2x and dx in equation I = sec 2 2 1 2 sec 2 = 2 1 2 sec 2 2 sec 2 tan 2 = 2 1 2 = 1 2 2 1 = 1 2 2 1 = 1 2 2 1 2 1 = 1 2 3 3 PuttingKeep breaking it down until you find something you can work with Let u=sec^2 and v=tan^2 and if that's still too much at this stage Let a=sec b=sec c=tan d=tan Differentiate a and b, use the product rule to find u;$$\int \sec^2x \tan^2x dx 2\int \sec^2x \tan^2x dx= tan^2x c, c\in\mathbb{R}$$ Note that once we have a side without an integral on it you need to include a constant of integration I have used $c$ The two expressions on the left hand side are the same so you can add them giving $$3\int \sec^2x \tan^2x dx= tan^2x c$$ So simply divide by 3 to get your answer



Integral 1 Tan2x Sec2x Cute766


Math Problems Simplifying With Trigonometry Identities And Then Integration
Free integral calculator solve indefinite, definite and multiple integrals with all the steps Type in any integral to get the solution, steps and graphIntegral of tan^2 (x)*sec (x) · 10) \(\displaystyle ∫\tan^2x\sec^2x\,dx\) Compute the following integrals using the guidelines for integrating powers of trigonometric functions Use a CAS to check the solutions (Note Some of the problems may be done using techniques of integration learned previously) 11) \(\displaystyle ∫\sin^3x\,dx\) Answer



1 Tan1 1 Tan2 1 Tan3 1 Tan45 2 N Brainly In Ryad Antonio Ebooks En Ligne Gratuit



Int Sec 2x Tan 2x 4 Dx Maths Questions
Find the Integral sec(2x)tan(2x) Let Then , so Rewrite using and Tap for more steps Let Find Tap for more steps Differentiate Differentiate using the chain rule, which states that is where and Since is constant with respect to , move out of the integralSee the answer See the answer See the answer done loading Show transcribed image textMy steps ∫ sec 3 ( 2 x) d x Let u = 2 x, then 1 2 d u = d x 1 2 ∫ sec 3
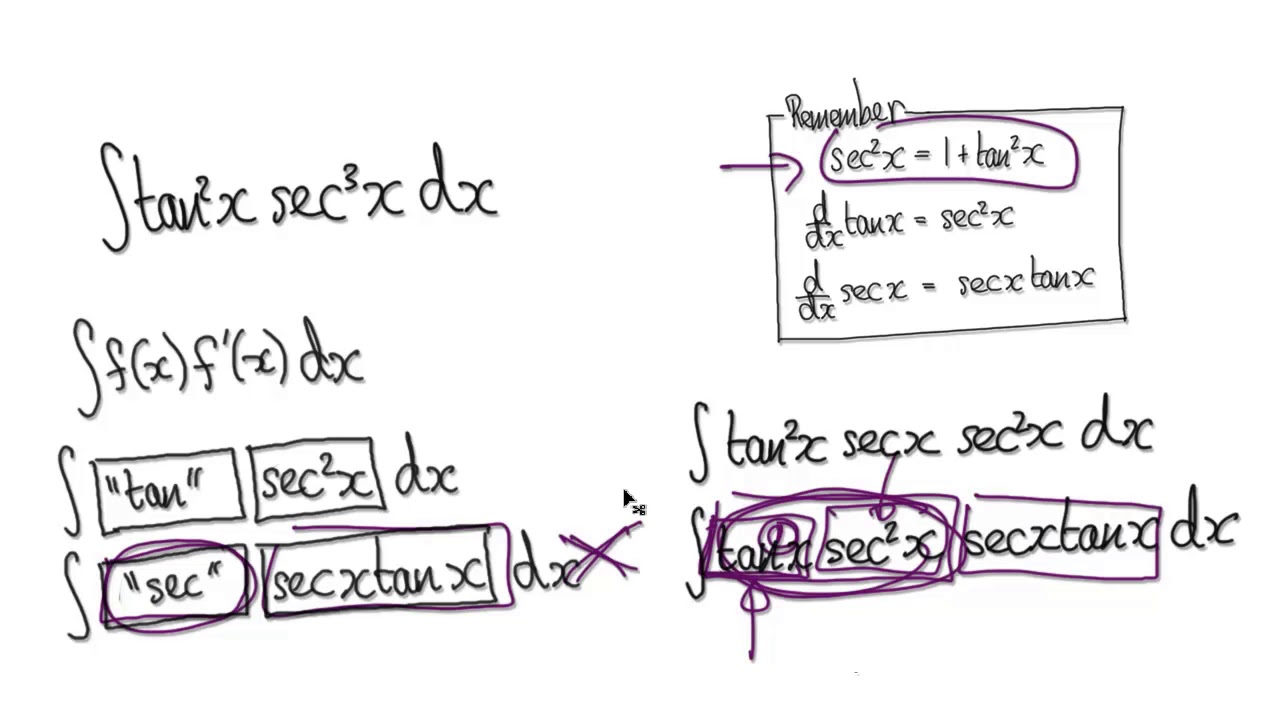


Video 34 6 Integrate Tan 2x Sec 3x Reduction Formula Practice Youtube



Solved Calculate The Given Indefinite Integral Integrate Chegg Com
This problem has been solved!{eq}\int \tan x \sec^2x dx {/eq} Integration by u Substitution We can find the indefinite integrals of some functions by applying the method of usubstitutionIntegral of sec^2x/(secx tanx)^n Functions involving trigonometric functions are useful as they are good at describing periodic behavior This section describes several techniques for finding antiderivatives of certain combinations of trigonometric functions
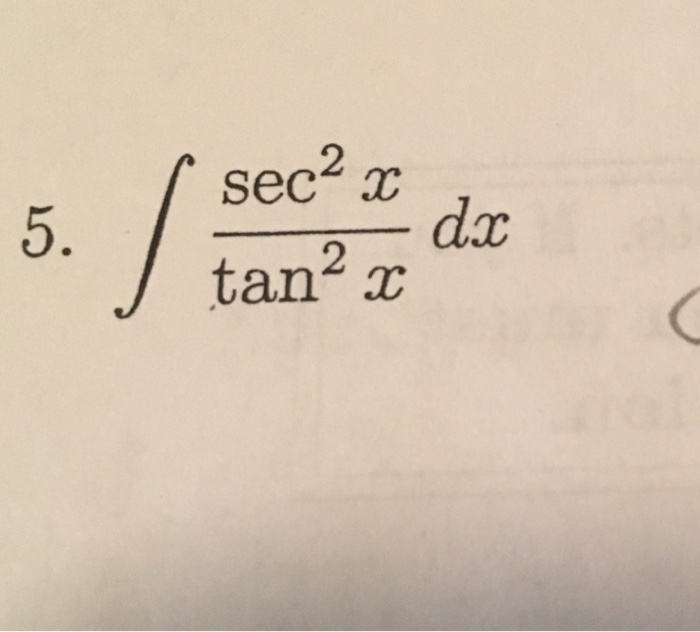


Solved Integral Sec 2 X Tan 2 X Dx Chegg Com



Evaluate I Inttanxsec 2x Sqrt 1 Tan 2x Dx Ii Intlogx
· So students this is final answer of integration of sec^x tan^x and as you can see in both of the method answer will be same is secx c Integration of sec^2x integration of secant squared x Let try to solving, in this case we solve the \( \int(sec^2x) dx \) Once again, I = \( \int(sec^2x) dx \) Now multiply tanx/tanx with the sec^2x And we get,



If Inte Secx Secxtanxf X Secxtanx Tan 2 X Dx E Secx F X C



Tan2x ただの悪魔の画像
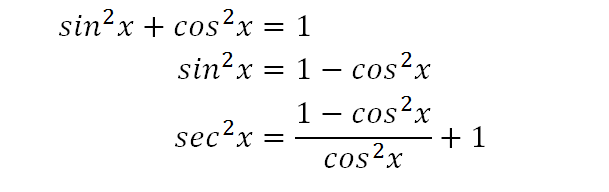


Integrate Sec 2x Method 2



Int Sec 2x 16 Tan 2x Dx



Integral De Tan X Sec 2 X Dx
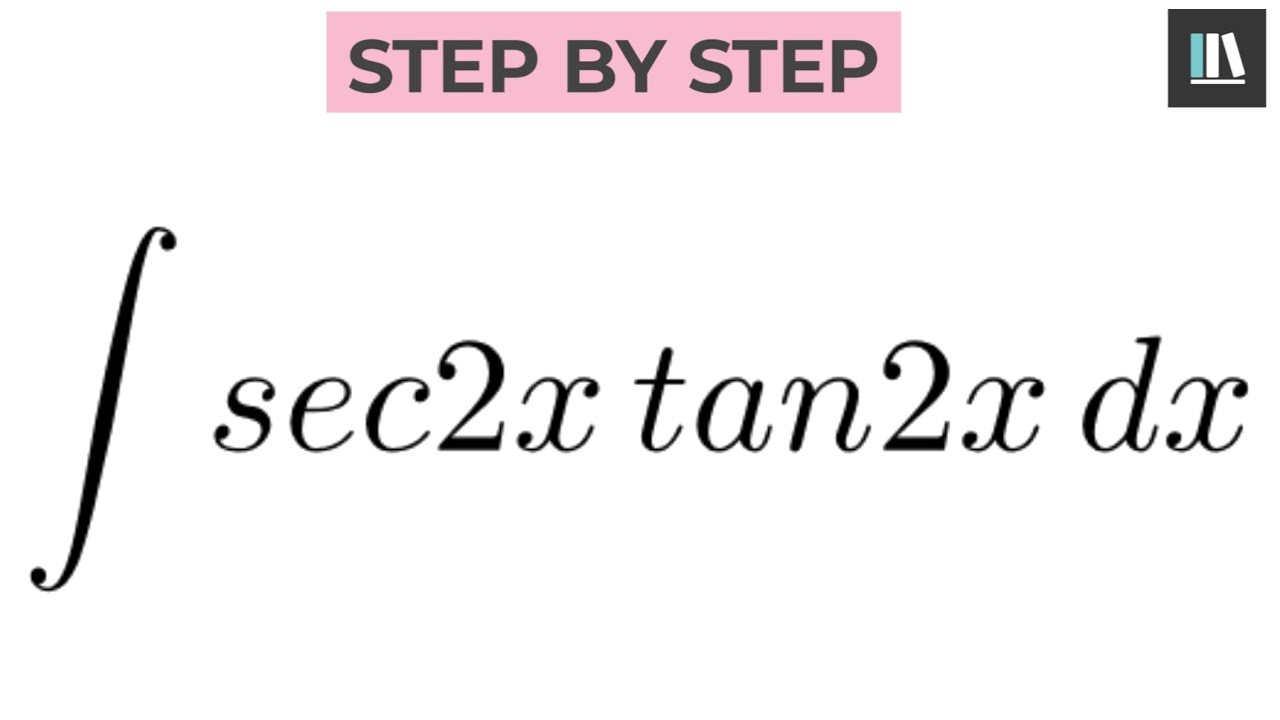


Integral Sec2x Tan2x Dx Youtube



What Is The Integration Of Tan 2x Solution Quora



Solved Evaluate Integral Tan 2 X Sec 2 X Dx Sec 3 X 3 C Chegg Com



The Value Of Int Tan 3 2x Sec 2x Dx Is Equal To Youtube



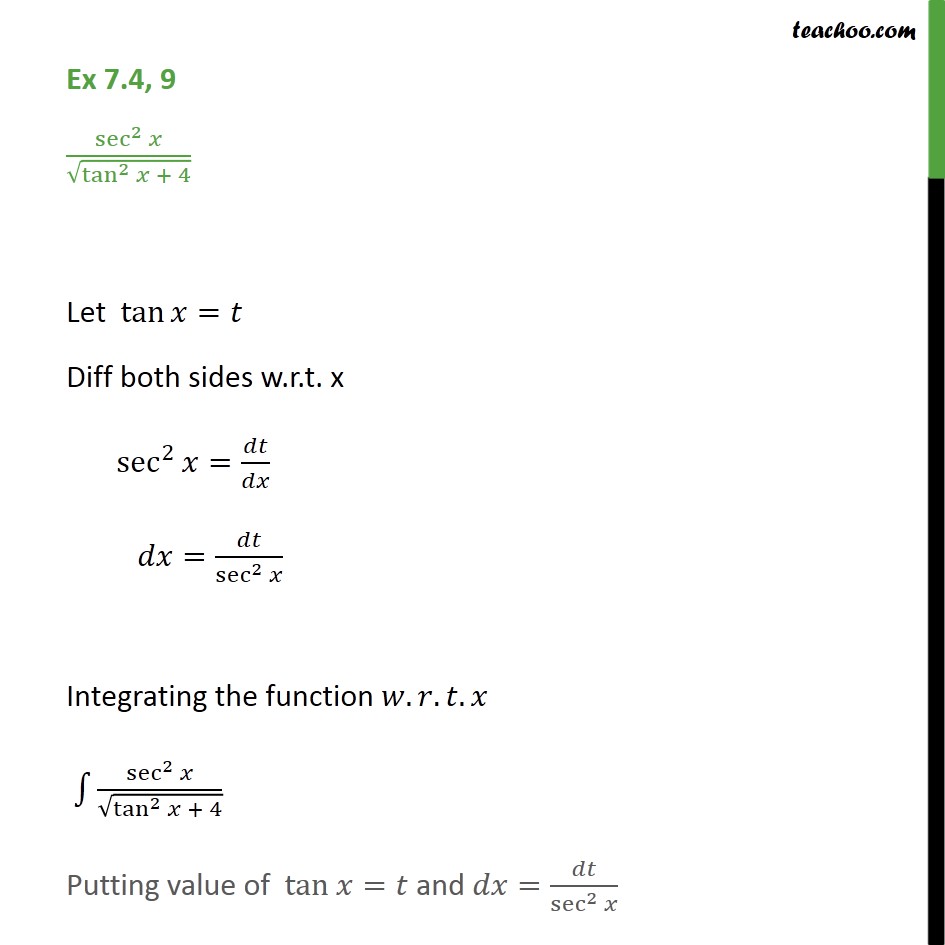
Ex 7 4 9 Integrate Sec 2 X Root Tan 2 X 4 Ncert Class 12
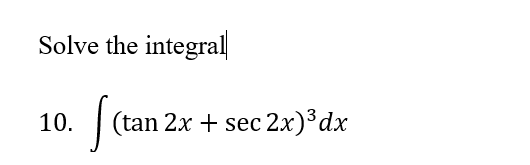


Answered Solve The Integral 10 Tan 2x Sec Bartleby



Integrate Tan 3 2x Sec2x Brainly In



Lw00plbaiwnh4m



7 2 47 Integral Of 1 Tan2x Sec2x Cute766


Integral Tan 2x Sec 2x 2 Brainly Co Id


Evaluate Sec 2 X 2 Tan X 3 Tan X Dx Sarthaks Econnect Largest Online Education Community


Integral Of Sec 6 X Tan 2 X Dx



Tan2x ただの悪魔の画像



Evaluate Int Tan 2xsec 2x 1 Tan 6x Dx



Evaluate Int 3tan 2x 2 Sec 2x Tan 3x 2tanx 5 Dx
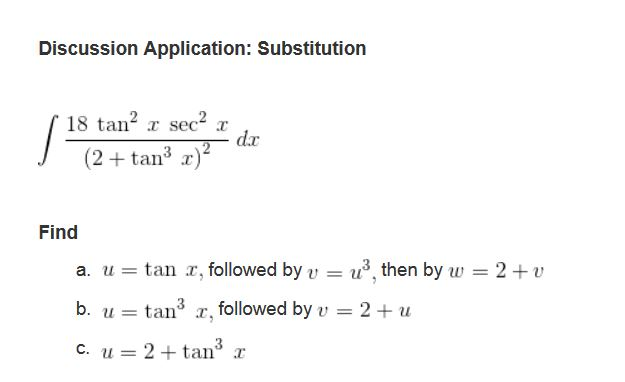


Solved Integral 18 Tan 2 X Sec 2 X 2 Tan 3 X 2 Dx Fin Chegg Com



Integration Of Sec 2 X Tan 3 X 4tanx Maths Integrals Meritnation Com



How To Integrate Math Sec 2x Tan 2x Dx Math Quora



Ongle Answer Cor Answer Correct Type 1 Tan X Cotx Then If Y Tanx Cotx A 2 Tan 2x Sec 2x B Tan 2x Sec 2x Vo 2 Tan 2x



Integral Tan 2x Sec 2x 2 Dx



Leslie Ruo S Blog Rusty Calculus


What Is The Integral Of Sec 2x Tan 2x Dx Brainly Com



Evaluate The Definite Integrals Int 0 Pi 4 2 Sec 2 X X 3 2 Dx Mathematics Shaalaa Com
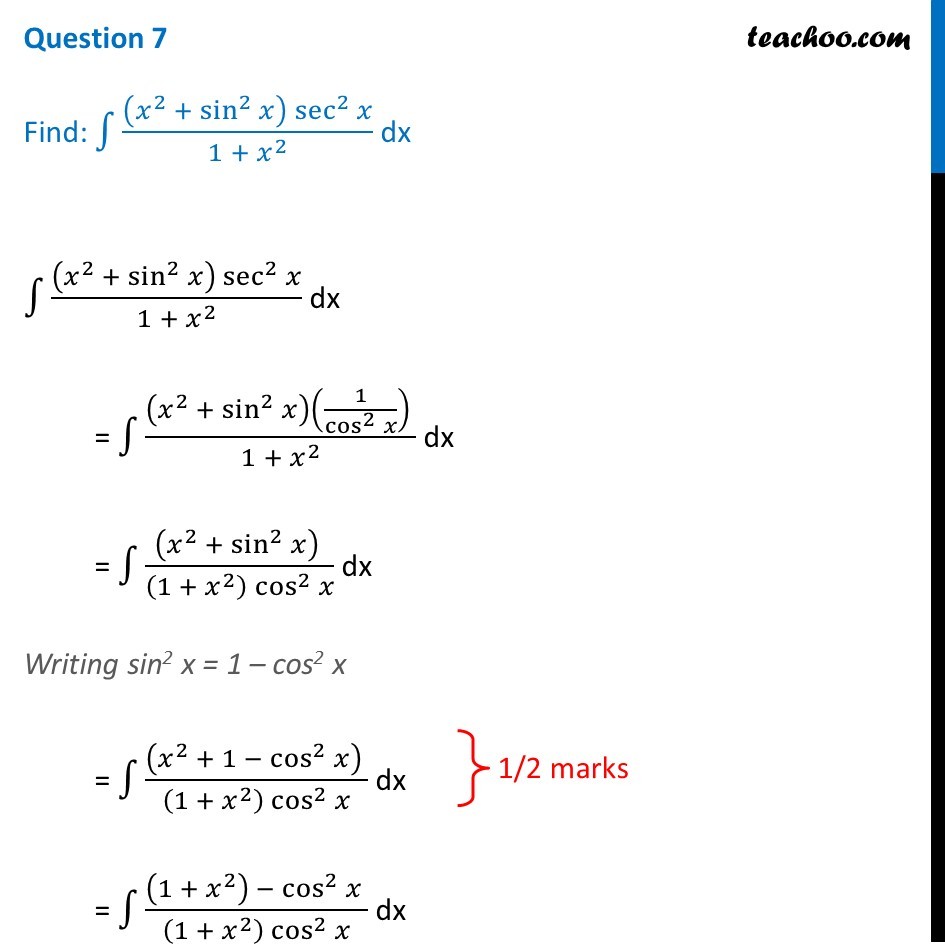


Find Integral X 2 Sin 2 X Sec 2 X 1 X 2 Teachoo



Integrate Sec 2x Method 1
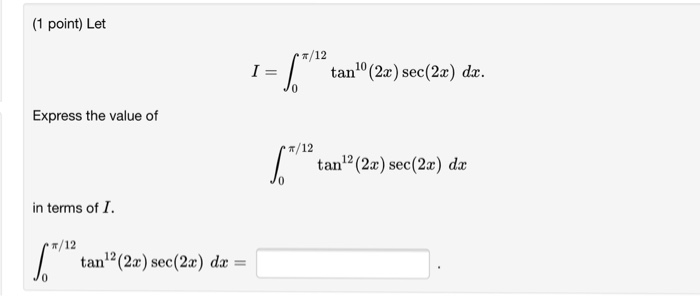


Solved Let I Integral From 0 To Pi 12 Of Tan 10 2x Sec Chegg Com



Answered If You Do Not Know What Substitution To Bartleby



Integral Of Sec 2x



Integration Of Tan 2 X Sec 2 X Youtube
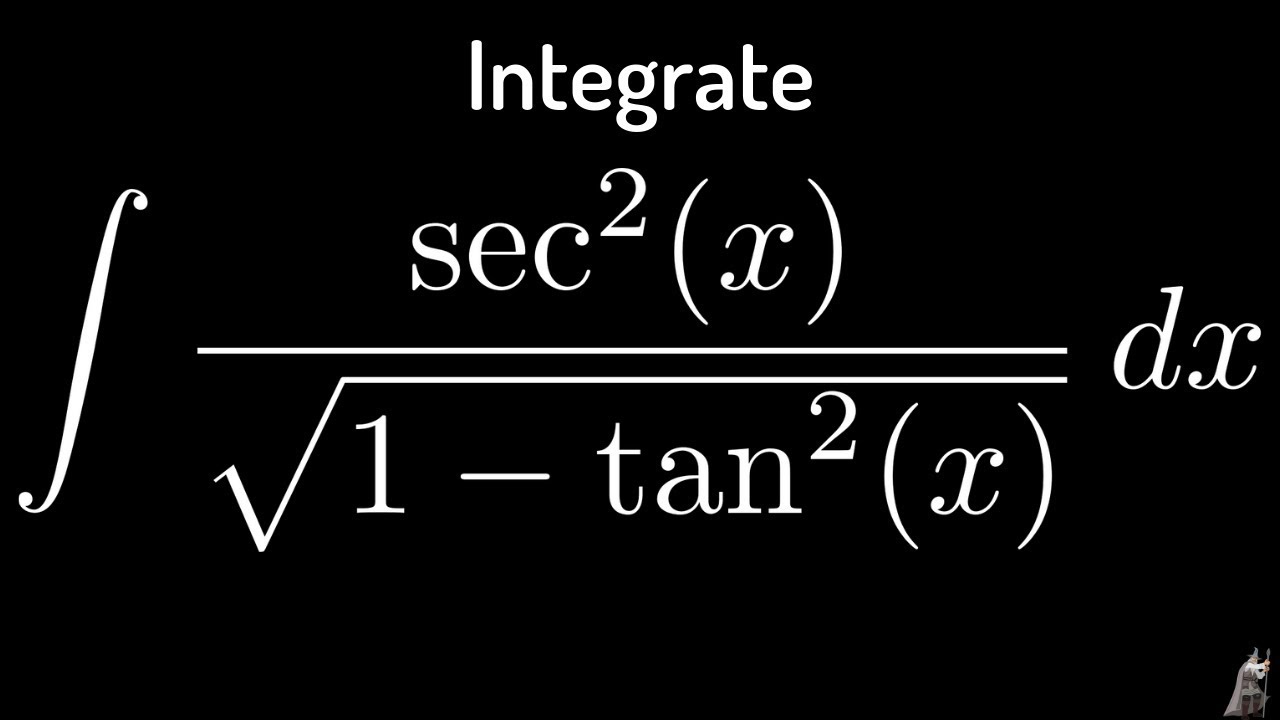


Integral Of Sec 2 X Sqrt 1 Tan 2 X Using The Arcsine Function Youtube



Ex 7 3 15 Integrate Tan3 2x Sec 2x Class 12 Ncert Ex 7 3


What Is The Integral Of Sec 4 2x Tan 3 2x Dx Quora



Integral Sec 2 X Tan 2 X Youtube Cute766
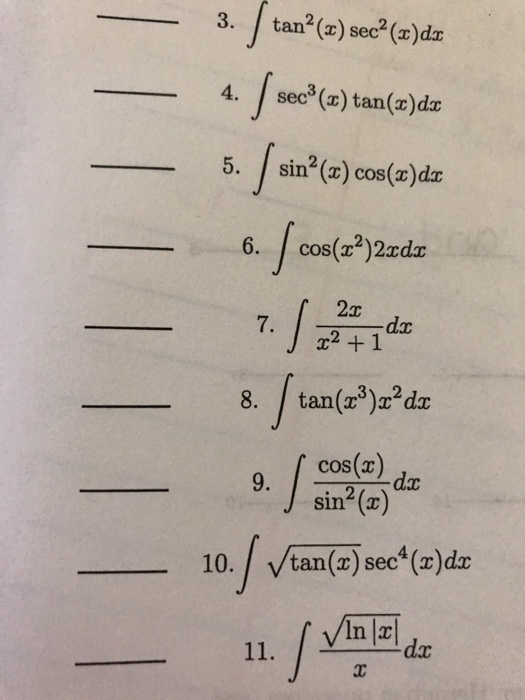


Solved Integral Tan 2 X Sec 2 X Dx Integral Sec 3 X Chegg Com



Integral Of Tan 2x Cot 2x 2 Calculus 1 Trig Integrals Calculus Mathematics Email Subject Lines


Integration Of Trigonometric Functions



Integral Tan 2 X Sec X Youtube



Calculus 7 2 22 Integral Of Tan 2x Sec 4x Youtube



How Do You Simplify Sec 4x Tan 4x Sec 2x Tan 2x Socratic


Ex 7 3 15 Integrate Tan3 2x Sec 2x Class 12 Ncert Ex 7 3


How To Integrate Math Sec 2x Tan 2x Dx Math Quora



What Is The Integral Of Sec X Sec 2x Quora



Solved Evaluate Integral Tan 6 2x Sec 4 2x Dx If We Chegg Com



Answered Find The Indefinite Integral Sec 2x Bartleby



Integral Of Tan 2x Sec 2x Dx



Integral 4 Tan 2x Sec 2x Dx Brainly Co Id



Derivative Of Sec 2x
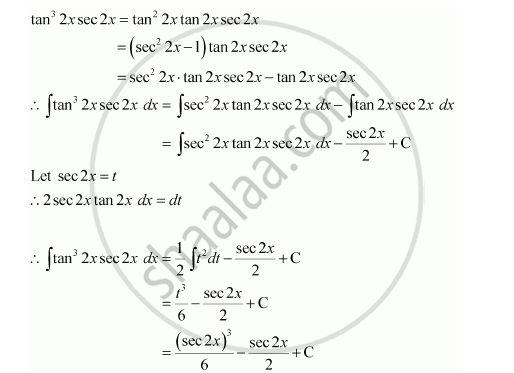


Find The Integrals Of The Functions Tan3 2x Sec 2x Mathematics Shaalaa Com



Integration Of Xsec 2x Tanx Dx Brainly In
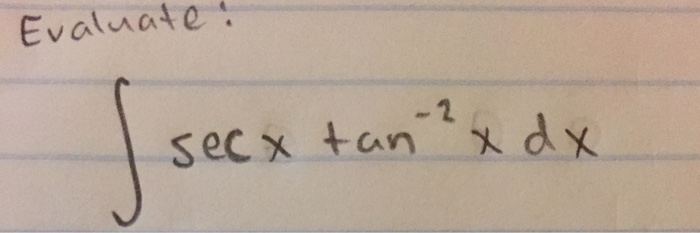


Solved Evaluate Integral Sec X Tan 2 X Dx Chegg Com



Examples Int Tan 2x Sec 4x Dx



Int Tan 2x Secx 1 Dx Youtube



Evaluating Trig Integral Int Tan 3x Sec 2x Dx Mathematics Stack Exchange


Integral Of Sec 6 X Tan 2 X Dx



Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo
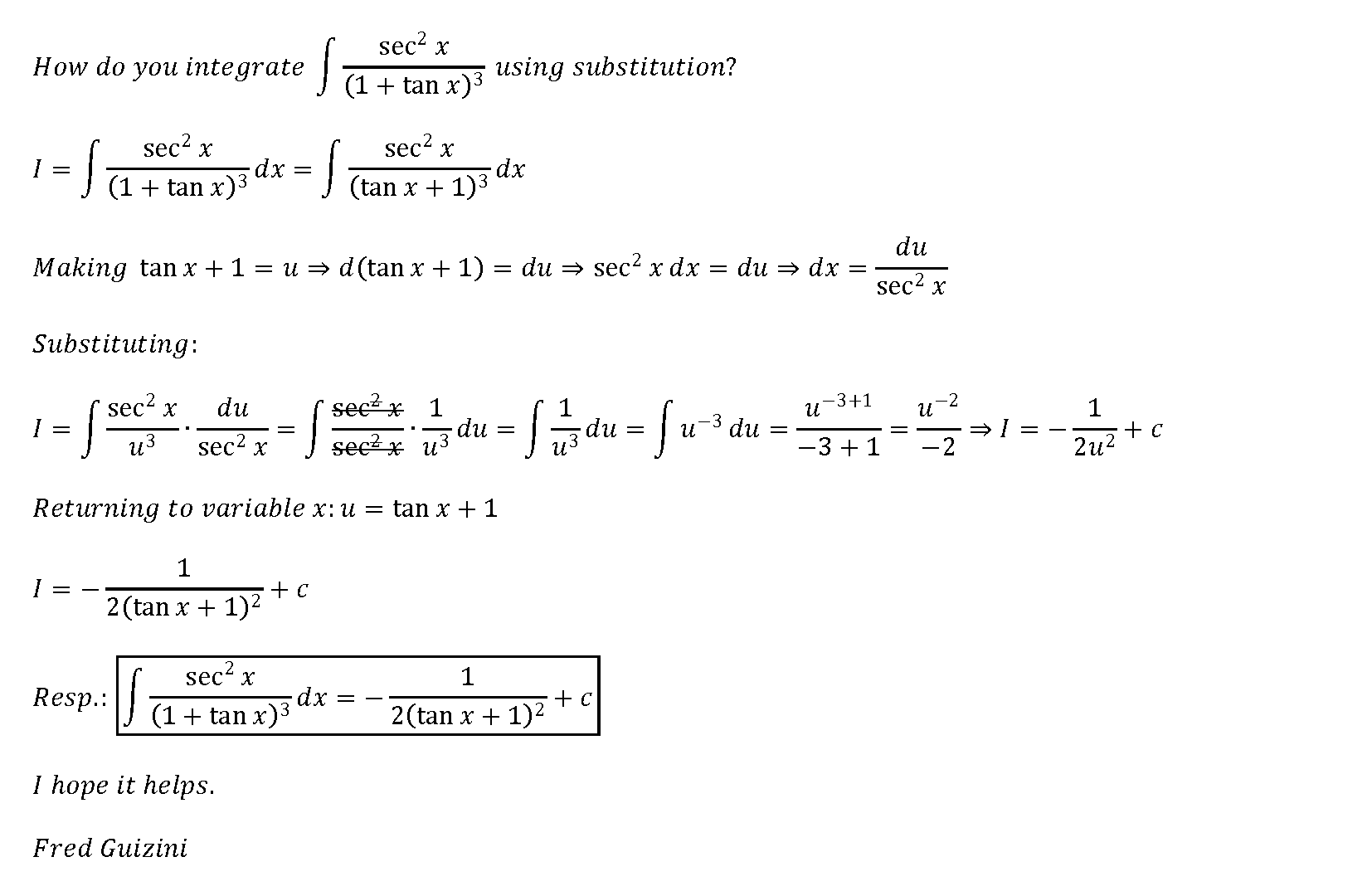


How Do You Integrate Int Sec 2x 1 Tanx 3 Using Substitution Socratic
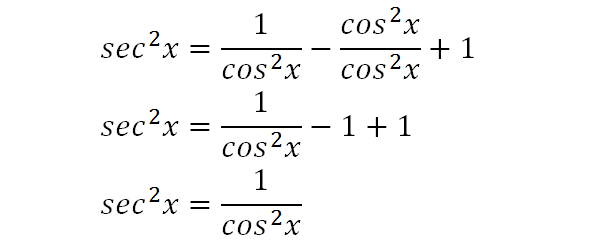


Integrate Sec 2x Method 2



If Tan 2 X Sec X A 0 Has Atleast One Solution Then Complete Set Of V Askiitians



How Do You Integrate 1 Tan2x Sec2x Dx Socratic



Can I Integrate Math Tan 2 X Sec 3 X Math Using The Substitution Quora


Integral Of Tan 4x Sec 2x Dx



Doc Integrate 1 Christian Galvez Academia Edu



Integrate Sec 2x Method 2


Evaluate Sec 2 X Tan 3 X 4 Tan X Dx Sarthaks Econnect Largest Online Education Community
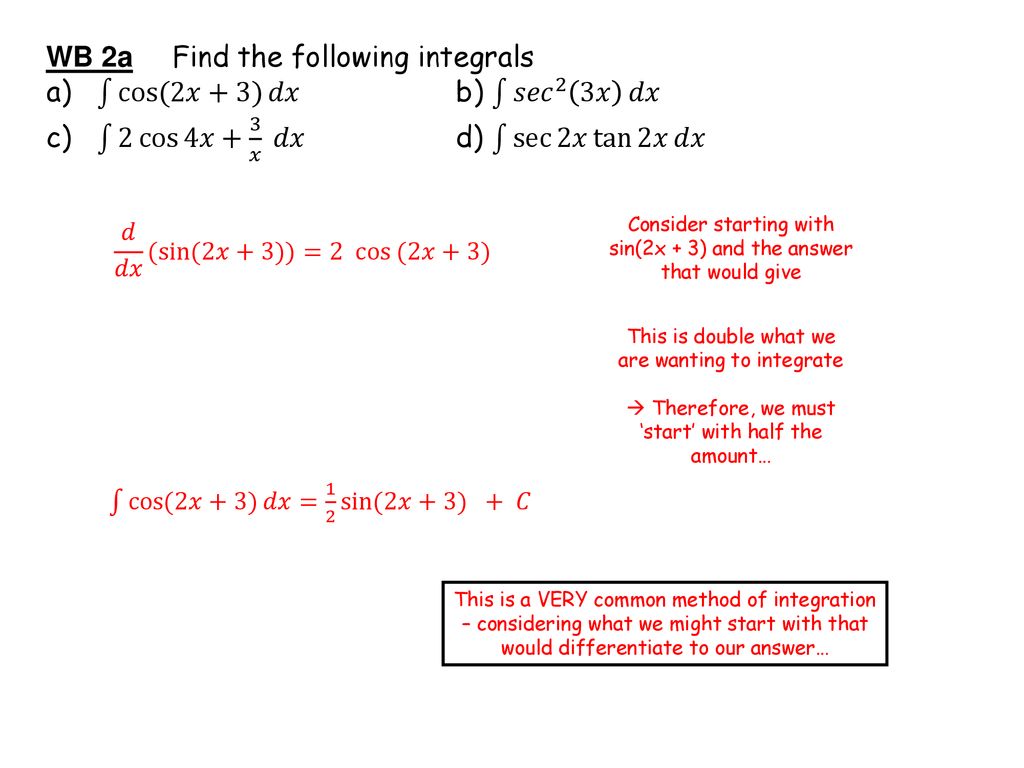


Integration Trig Identities Ppt Download



Ex 7 2 21 Integrate Tan2 2x 3 Class 12 Cbse Ex 7 2
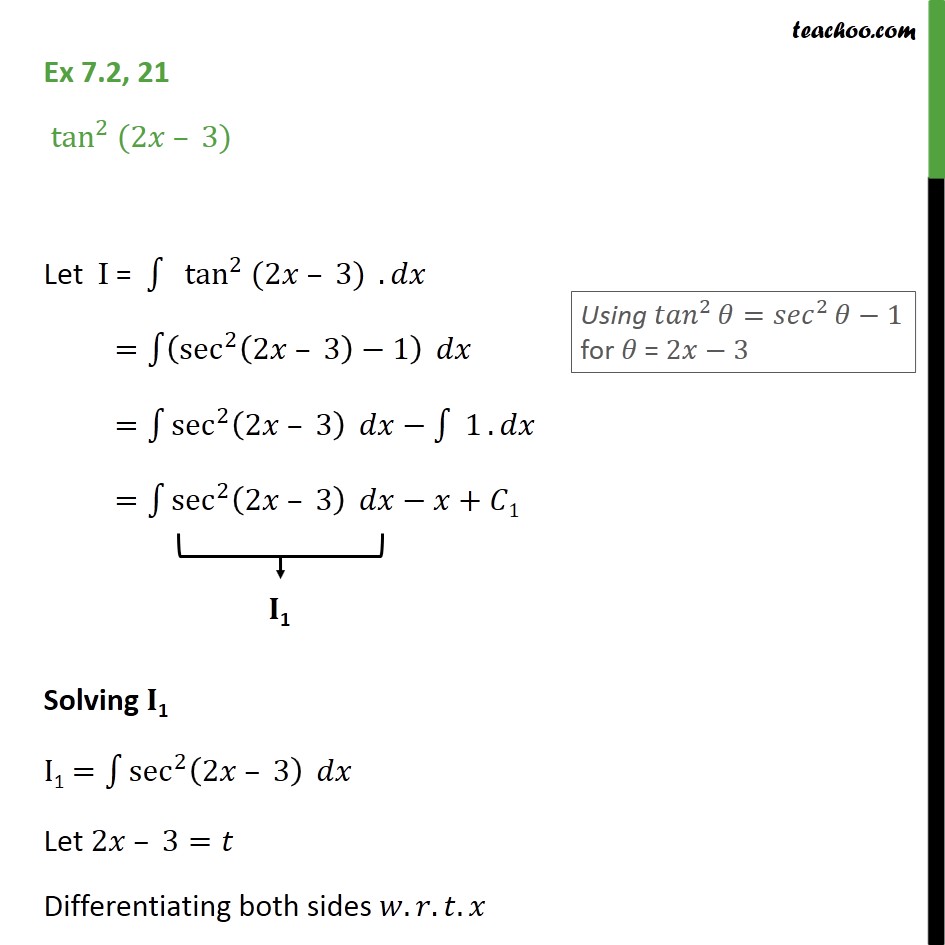


Ex 7 2 21 Integrate Tan2 2x 3 Class 12 Cbse Ex 7 2



Integrating Tan 2 2x Youtube


Find The Integrals Of The Functions Tan 3 2x Sec 2x Sarthaks Econnect Largest Online Education Community



Evaluate Int Sec 2x 1 Tan 2x Dx



0 件のコメント:
コメントを投稿